Tangential or tangential acceleration. Acceleration - average, instantaneous, tangential, normal, total Tangential acceleration characterizes the change in speed

The basic formulas of the kinematics of a material point, their derivation and presentation of the theory are given.

Content

See also: An example of solving a problem (coordinate method of specifying the movement of a point)

Basic formulas for the kinematics of a material point

Let us present the basic formulas of the kinematics of a material point. After which we will give their conclusion and presentation of the theory.

Radius vector of the material point M in the rectangular coordinate system Oxyz:
,
where are unit vectors (orts) in the direction of the x, y, z axes.

Point speed:
;
.
.
Unit vector in the direction tangent to the trajectory of a point:
.

Acceleration point:
;
;
;
; ;

Tangential (tangential) acceleration:
;
;
.

Normal acceleration:
;
;
.

Unit vector directed towards the center of curvature of the point's trajectory (along the main normal):
.

Radius vector and point trajectory

Let's consider the movement of the material point M. Let us choose a fixed rectangular coordinate system Oxyz with a center at some fixed point O. Then the position of point M is uniquely determined by its coordinates (x, y, z). These coordinates are components of the radius vector of the material point.

The radius vector of a point M is a vector drawn from the origin of a fixed coordinate system O to a point M.
,
where are unit vectors in the direction of the x, y, z axes.

When a point moves, the coordinates change over time. That is, they are functions of time. Then the system of equations
(1)
can be thought of as the equation of a curve defined by parametric equations. Such a curve is the trajectory of a point.

The trajectory of a material point is the line along which the point moves.

If the point moves in a plane, then the axes and coordinate systems can be selected so that they lie in this plane. Then the trajectory is determined by two equations

In some cases, time can be eliminated from these equations. Then the trajectory equation will have the form:
,
where is some function. This dependency contains only the variables and . It does not contain the parameter.

Velocity of a material point

The speed of a material point is the derivative of its radius vector with respect to time.

According to the definition of speed and the definition of derivative:

In mechanics, derivatives with respect to time are denoted by a dot above the symbol. Let's substitute here the expression for the radius vector:
,
where we have clearly indicated the dependence of coordinates on time. We get:

,
Where
,
,

- projections of velocity on the coordinate axes. They are obtained by differentiating the components of the radius vector with respect to time
.

Thus
.
Speed Module:
.

Tangent to the path

From a mathematical point of view, the system of equations (1) can be considered as an equation of a line (curve) defined by parametric equations. Time, in this consideration, plays the role of a parameter. From the course of mathematical analysis it is known that the direction vector for the tangent to this curve has the components:
.
But these are the components of the point’s velocity vector. That is the velocity of the material point is directed tangentially to the trajectory.

All this can be demonstrated directly. Let at the moment of time the point be in a position with the radius vector (see figure). And at the moment of time - in position with the radius vector. Let's draw a straight line through the points. By definition, a tangent is a straight line to which the straight line tends as .
Let us introduce the following notation:
;
;
.
Then the vector is directed along the straight line.

When tending, the straight line tends to the tangent, and the vector tends to the speed of the point at the moment of time:
.
Since the vector is directed along the straight line, and the straight line at , the velocity vector is directed along the tangent.
That is, the velocity vector of a material point is directed along the tangent to the trajectory.

Let's introduce tangent direction vector of unit length:
.
Let us show that the length of this vector is equal to one. Indeed, since
, That:
.

Then the speed vector of the point can be represented as:
.

Acceleration of a material point

The acceleration of a material point is the derivative of its speed with respect to time.

Similar to the previous one, we obtain the components of acceleration (projections of acceleration on the coordinate axes):
;
;
;
.
Acceleration module:
.

Tangential (tangent) and normal acceleration

Now consider the question of the direction of the acceleration vector with respect to the trajectory. To do this we apply the formula:
.
We differentiate it with respect to time using the product differentiation rule:
.

The vector is directed tangentially to the trajectory. In which direction is its time derivative directed?

To answer this question, we use the fact that the length of the vector is constant and equal to unity. Then the square of its length is also equal to one:
.
Here and below, two vectors in parentheses denote the scalar product of vectors. Let's differentiate the last equation with respect to time:
;
;
.
Since the scalar product of vectors and is equal to zero, these vectors are perpendicular to each other. Since the vector is directed tangent to the trajectory, the vector is perpendicular to the tangent.

The first component is called tangential or tangential acceleration:
.
The second component is called normal acceleration:
.
Then the total acceleration is:
(2) .
This formula represents the decomposition of acceleration into two mutually perpendicular components - tangent to the trajectory and perpendicular to the tangent.

Since then
(3) .

Tangential (tangential) acceleration

Let's multiply both sides of the equation (2) scalar to :
.
Because , then . Then
;
.
Here we put:
.
From this we can see that the tangential acceleration is equal to the projection of the total acceleration onto the direction of the tangent to the trajectory or, which is the same, onto the direction of the point’s velocity.

Tangential (tangential) acceleration of a material point is the projection of its total acceleration onto the direction of the tangent to the trajectory (or to the direction of velocity).

We use the symbol to denote the tangential acceleration vector directed along the tangent to the trajectory. Then is a scalar quantity equal to the projection of the total acceleration onto the direction of the tangent. It can be both positive and negative.

Substituting , we have:
.

Let's put it into the formula:
.
Then:
.
That is, the tangential acceleration is equal to the time derivative of the absolute velocity of the point. Thus, tangential acceleration leads to a change in the absolute value of the point's speed. As the speed increases, the tangential acceleration is positive (or directed along the speed). As the speed decreases, the tangential acceleration is negative (or in the opposite direction to the speed).

Now let's examine the vector.

Consider a unit vector tangent to the trajectory. Let's place its origin at the origin of the coordinate system. Then the end of the vector will be on a sphere of unit radius. When a material point moves, the end of the vector will move along this sphere. That is, it will rotate around its origin. Let be the instantaneous angular velocity of rotation of the vector at the moment of time . Then its derivative is the speed of movement of the end of the vector. It is directed perpendicular to the vector. Let's apply the formula for rotating motion. Vector module:
.

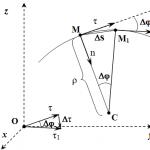
Now consider the position of the point for two close moments in time. Let the point be in position at the moment of time, and in position at the moment of time. Let and be unit vectors directed tangentially to the trajectory at these points. Through the points and we draw planes perpendicular to the vectors and . Let be a straight line formed by the intersection of these planes. From a point we lower a perpendicular to a straight line. If the positions of the points are close enough, then the movement of the point can be considered as rotation along a circle of radius around the axis, which will be the instantaneous axis of rotation of the material point. Since the vectors and are perpendicular to the planes and, the angle between these planes is equal to the angle between the vectors and. Then the instantaneous speed of rotation of the point around the axis is equal to the instantaneous speed of rotation of the vector:
.
Here is the distance between points and .

Thus we found the modulus of the time derivative of the vector:
.
As we indicated earlier, the vector is perpendicular to the vector. From the above reasoning it is clear that it is directed towards the instantaneous center of curvature of the trajectory. This direction is called the principal normal.

Normal acceleration

Normal acceleration

directed along the vector. As we found out, this vector is directed perpendicular to the tangent, towards the instantaneous center of curvature of the trajectory.
Let be a unit vector directed from the material point to the instantaneous center of curvature of the trajectory (along the main normal). Then
;
.
Since both vectors have the same direction - towards the center of curvature of the trajectory, then
.

From the formula (2) we have:
(4) .
From the formula (3) we find the normal acceleration module:
.

Let's multiply both sides of the equation (2) scalar to :
(2) .
.
Because , then . Then
;
.
This shows that the modulus of normal acceleration is equal to the projection of the total acceleration onto the direction of the main normal.

The normal acceleration of a material point is the projection of its total acceleration onto the direction perpendicular to the tangent to the trajectory.

Let's substitute . Then
.
That is, normal acceleration causes a change in the direction of the speed of a point, and it is related to the radius of curvature of the trajectory.

From here you can find the radius of curvature of the trajectory:
.

And in conclusion, we note that the formula (4) can be rewritten as follows:
.
Here we have applied the formula for the cross product of three vectors:
,
which they framed
.

So we got:
;
.
Let's equate the modules of the left and right parts:
.
But the vectors are also mutually perpendicular. That's why
.
Then
.
This is a well-known formula from differential geometry for the curvature of a curve.

Normal acceleration

.Normal acceleration – vector physical quantity characterizing the change in the direction of speed, numerically equal to the ratio of the square of the speed to the radius of curvature of the trajectory, directed along the radius of curvature to the center of curvature:

like vectors

And

directed at right angles, then (Fig. 1. 17)

, (1.2.9)

5.Angular acceleration – a vector physical quantity characterizing the change in angular velocity, numerically equal to the first derivative of the angular velocity with respect to time and directed along the axis of rotation in the same direction as the angular velocity if the speed increases, and opposite to it if it decreases.

Insert formula (1.2.10)

SI:

Full acceleration

(linear)

Since we are limited to considering rotation around a fixed axis, angular acceleration is not divided into components like linear acceleration.

Angular acceleration

Relationship between angular characteristics

rotating body and linear

characteristics of the movement of its individual points

SI:

Let's consider one of the points of a rotating body, which is located at a distance R from the axis of rotation, that is, it moves along a circle of radius R (Fig. 1.18).

After time has passed
point A will move to position A 1, having covered the distance
, the radius vector will rotate by an angle
. Central angle subtended by an arc
, in radian measure, is equal to the ratio of the length of the arc to the radius of curvature of this arc:

This remains true for an infinitesimal time interval
:
. Further, using the definitions, it is easy to obtain:

; (1.2.11)

Relationship between linear and angular characteristics

; (1.2.12)

. (1.2.13)

1.1.2. Classification of movements. Kinematic laws

We will call kinematic laws laws that express changes in the kinematic characteristics of movement over time:

Law of the way
or
;

Law of Speed
or
;

Law of Acceleration
or
.

Acceleration

Acceleration of a racing car at the start is 4-5 m/s 2

Acceleration of a jet plane upon landing

6-8 m/c 2

Gravity acceleration near the surface of the Sun 274 m/c 2

Acceleration of a projectile in a gun barrel 10 5 m/c 2

The most informative characteristic of movement is acceleration, so it is used as the basis for classifying movements.

Normal acceleration carries information about a change in the direction of speed, that is, about the features of the trajectory of movement:

- motion is linear (the direction of speed does not change);

- curvilinear movement.

Tangential acceleration determines the nature of the change in velocity modulus over time. On this basis, it is customary to distinguish the following types of movement:

- uniform movement (the absolute value of the speed does not change);

- accelerated movement

- uneven - (speed increases)

new movement
-slow motion

speed (speed decreases).

The simplest special cases of uneven motion are movements in which

- tangential acceleration does not depend on time, remains constant during movement - uniformly variable movement (uniformly accelerated or uniformly decelerated);

or
- tangential acceleration changes over time according to the law of sine or cosine - harmonic oscillatory motion (for example, a weight on a spring).

Likewise for rotational motion:

- uniform rotation;

- uneven rotation

Write types of movement more compactly

-uniformly accelerated

rotation

- slow-

no rotation;

- equal-

belt rotation

Torsional vibrations (for example, trifilar suspension - a disk suspended on three elastic threads and oscillating in the horizontal plane).

If one of the kinematic laws is known in analytical form, then others can be found, and two types of problems are possible:

Type I – according to a given path law
or
find the speed law
or
and the law of acceleration
or
;

Type II – according to a given acceleration law
or
find the speed law
or
and the law of the way
or
.

These problems are mutually inverse and are solved using inverse mathematical operations. The first type of problem is solved on the basis of definitions, that is, by applying the operation of differentiation.

- set

- ?

- ?
.

The second type of problem is solved by integration. If the speed is the first derivative of the path with respect to time, then the path with respect to the speed can be found as an antiderivative. Similarly: acceleration is the derivative of speed with respect to time, then speed with respect to acceleration is antiderivative. Mathematically, these actions look like this:

- increment of path over an infinitesimal period of time
. For a finite interval from before integrate:
. According to the rules of integration
. To take the integral on the right side, you need to know the form of the rate law, that is
. Finally, to find the position of the body on the trajectory at an arbitrary moment in time, we obtain:

, where (1.2.14)

- change in speed over an infinitesimal period of time
.

For a finite interval from before :

Tangential acceleration characterizes the change in speed in absolute value (magnitude) and is directed tangentially to the trajectory:

Where  derivative of the velocity module,  unit tangent vector, coinciding in direction with the speed.

Normal acceleration characterizes the change in speed in direction and is directed along the radius of curvature to the center of curvature of the trajectory at a given point:

where R is the radius of curvature of the trajectory,  unit normal vector.

The magnitude of the acceleration vector can be found using the formula

1.3. The main task of kinematics

The main task of kinematics is to find the law of motion of a material point. For this, the following relationships are used:

;
;
;
;

Special cases of rectilinear motion:

1) uniform linear motion: ;

2) uniform linear motion:
.

1.4. Rotational motion and its kinematic characteristics

During rotational motion, all points of the body move in circles, the centers of which lie on the same straight line, called the axis of rotation. To characterize the rotational motion, the following kinematic characteristics are introduced (Fig. 3).

Angular movement
 vector numerically equal to the angle of rotation of the body
during
and directed along the axis of rotation so that, looking along it, the rotation of the body is observed to occur clockwise.

Angular velocity  characterizes the speed and direction of rotation of the body, is equal to the derivative of the angle of rotation with respect to time and is directed along the axis of rotation as angular displacement.

P For rotational motion, the following formulas are valid:

;
;
.

Angular acceleration characterizes the rate of change in angular velocity over time, equal to the first derivative of the angular velocity and directed along the axis of rotation:

;
;
.

Addiction
expresses the law of body rotation.

With uniform rotation:  = 0,  = const,  = t.

With uniform rotation:  = const,
,
.

To characterize uniform rotational motion, the rotation period and rotation frequency are used.

Rotation period T is the time of one revolution of a body rotating at a constant angular velocity.

Rotation frequency – the number of revolutions made by the body per unit of time.

Angular velocity can be expressed as follows:

Relationship between angular and linear kinematic characteristics (Fig. 4):

2. Dynamics of translational and rotational movements

Newton's laws Newton's first law: every body is in a state of rest or uniform rectilinear motion until the influence of other bodies takes it out of this state.

Bodies that are not subject to external influences are called free bodies. The reference system associated with a free body is called an inertial reference system (IRS). In relation to it, any free body will move uniformly and rectilinearly or be at rest. From the relativity of motion it follows that a reference system moving uniformly and rectilinearly with respect to the ISO is also an ISO. ISOs play an important role in all branches of physics. This is due to Einstein's principle of relativity, according to which the mathematical form of any physical law must have the same form in all inertial frames of reference.

The basic concepts used in the dynamics of translational motion include force, body mass, and momentum of the body (system of bodies).

By force is a vector physical quantity that is a measure of the mechanical action of one body on another. Mechanical action occurs both through direct contact of interacting bodies (friction, support reaction, weight, etc.), and through force field existing in space (gravity, Coulomb forces, etc.). Force characterized by module, direction and point of application.

Simultaneous action of several forces on the body ,,...,can be replaced by the action of the resultant (resultant) force :

=++...+=.

Mass of a body is a scalar quantity that is a measure inertia bodies. Under inertia refers to the property of material bodies to maintain their speed unchanged in the absence of external influences and change it gradually (i.e. with finite acceleration) under the influence of force.

Impulse body (material point) is a vector physical quantity equal to the product of the body’s mass and its speed:
.

The momentum of a system of material points is equal to the vector sum of the momentum of the points that make up the system:
.

Newton's second law: the rate of change of momentum of a body is equal to the force acting on it:

If the mass of the body remains constant, then the acceleration acquired by the body relative to the inertial frame of reference is directly proportional to the force acting on it and inversely proportional to the mass of the body:

i.e., it is equal to the first derivative with respect to time of the speed modulus, thereby determining the rate of change of speed in modulus.

The second component of acceleration, equal to

called normal component of acceleration and is directed along the normal to the trajectory to the center of its curvature (therefore it is also called centripetal acceleration).

So, tangential acceleration component characterizes speed of change of speed modulo(directed tangentially to the trajectory), and normal acceleration component - speed of change of speed in direction(directed towards the center of curvature of the trajectory).

Depending on the tangential and normal components of acceleration, motion can be classified as follows:

1) , and n = 0 - rectilinear uniform motion;

2) , and n = 0 - rectilinear uniform motion. With this type of movement

If the initial time t 1 =0, and the initial speed v 1 =v 0 , then, denoting t 2 =t And v 2 =v, we get where from

By integrating this formula over the range from zero to an arbitrary point in time t, we find that the length of the path traveled by a point in the case of uniformly variable motion

· 3) , and n = 0 - linear motion with variable acceleration;

· 4) , and n = const. When the speed does not change in absolute value, but changes in direction. From the formula a n =v 2 /r it follows that the radius of curvature must be constant. Therefore, the circular motion is uniform;

· 5) , - uniform curvilinear movement;

· 6) , - curvilinear uniform motion;

· 7) , - curvilinear movement with variable acceleration.

2) A rigid body moving in three-dimensional space can have a maximum of six degrees of freedom: three translational and three rotational

Elementary angular displacement is a vector directed along the axis according to the rule of the right screw and numerically equal to the angle

Angular velocity is a vector quantity equal to the first derivative of the angle of rotation of a body with respect to time:

The unit is radian per second (rad/s).

Angular acceleration is a vector quantity equal to the first derivative of the angular velocity with respect to time:

When a body rotates around a fixed axis, the angular acceleration vector is directed along the rotation axis towards the vector of the elementary increment of angular velocity. When the movement is accelerated, the vector is codirectional to the vector (Fig. 8), when it is slow, it is opposite to it (Fig. 9).

Tangential component of acceleration

Normal component of acceleration

When a point moves along a curve, the linear speed is directed

tangent to the curve and modulo equal to the product

angular velocity to the radius of curvature of the curve. (connection)

3) Newton's first law: every material point (body) maintains a state of rest or uniform rectilinear motion until the influence of other bodies forces it to change this state. The desire of a body to maintain a state of rest or uniform rectilinear motion is called inertia. Therefore, Newton's first law is also called law of inertia.

Mechanical motion is relative, and its nature depends on the frame of reference. Newton's first law is not satisfied in every frame of reference, and those systems in relation to which it is satisfied are called inertial reference systems. An inertial reference system is a reference system relative to which the material point, free from external influences, either at rest or moving uniformly and in a straight line. Newton's first law states the existence of inertial frames of reference.

Newton's second law - the basic law of the dynamics of translational motion - answers the question of how the mechanical motion of a material point (body) changes under the influence of forces applied to it.

Weight body - a physical quantity that is one of the main characteristics of matter, determining its inertial ( inert mass) and gravitational ( gravitational mass) properties. At present, it can be considered proven that the inertial and gravitational masses are equal to each other (with an accuracy of at least 10–12 of their values).

So, force is a vector quantity that is a measure of the mechanical impact on a body from other bodies or fields, as a result of which the body acquires acceleration or changes its shape and size.

Vector quantity

numerically equal to the product of the mass of a material point and its speed and having the direction of speed is called impulse (amount of movement) this material point.

Substituting (6.6) into (6.5), we get

This expression - a more general formulation of Newton's second law: the rate of change of momentum of a material point is equal to the force acting on it. The expression is called equation of motion of a material point.

Newton's third law

The interaction between material points (bodies) is determined Newton's third law: every action of material points (bodies) on each other is in the nature of interaction; the forces with which material points act on each other are always equal in magnitude, oppositely directed and act along the straight line connecting these points:

F 12 = – F 21, (7.1)

where F 12 is the force acting on the first material point from the second;

F 21 - force acting on the second material point from the first. These forces are applied to different material points (bodies), always act in pairs and are forces of the same nature.

Newton's third law allows for the transition from dynamics separate material point to dynamics systems material points. This follows from the fact that for a system of material points, the interaction is reduced to the forces of pairwise interaction between material points.

Elastic force is a force that arises during deformation of a body and counteracts this deformation.

In the case of elastic deformations, it is potential. The elastic force is of an electromagnetic nature, being a macroscopic manifestation of intermolecular interaction. In the simplest case of tension/compression of a body, the elastic force is directed opposite to the displacement of the particles of the body, perpendicular to the surface.

The force vector is opposite to the direction of deformation of the body (displacement of its molecules).

Hooke's law

In the simplest case of one-dimensional small elastic deformations, the formula for the elastic force has the form: where k is the rigidity of the body, x is the magnitude of the deformation.

GRAVITY, a force P acting on any body located near the earth's surface, and defined as the geometric sum of the Earth's gravitational force F and the centrifugal force of inertia Q, taking into account the effect of the Earth's daily rotation. The direction of gravity is vertical at a given point on the earth's surface.

existence friction forces, which prevents sliding of contacting bodies relative to each other. Friction forces depend on the relative velocities of the bodies.

There are external (dry) and internal (liquid or viscous) friction. External friction is called friction that occurs in the plane of contact of two contacting bodies during their relative movement. If the bodies in contact are motionless relative to each other, they speak of static friction, but if there is a relative movement of these bodies, then, depending on the nature of their relative motion, they speak of sliding friction, rolling or spinning.

Internal friction is called friction between parts of the same body, for example between different layers of liquid or gas, the speed of which varies from layer to layer. Unlike external friction, there is no static friction here. If bodies slide relative to each other and are separated by a layer of viscous liquid (lubricant), then friction occurs in the lubricant layer. In this case they talk about hydrodynamic friction(the lubricant layer is quite thick) and boundary friction (the thickness of the lubricant layer is »0.1 microns or less).

experimentally established the following law: sliding friction force F tr is proportional to force N normal pressure with which one body acts on another:

F tr = f N ,

Where f- sliding friction coefficient, depending on the properties of the contacting surfaces.

f = tga 0.

Thus, the friction coefficient is equal to the tangent of the angle a 0 at which the body begins to slide along the inclined plane.

For smooth surfaces, intermolecular attraction begins to play a certain role. For them it is applied sliding friction law

F tr = f ist ( N + Sp 0) ,

Where R 0 - additional pressure caused by intermolecular attractive forces, which quickly decrease with increasing distance between particles; S- contact area between bodies; f ist - true coefficient of sliding friction.

The rolling friction force is determined according to the law established by Coulomb:

F tr = f To N/r , (8.1)

Where r- radius of the rolling body; f k - rolling friction coefficient, having the dimension dim f k =L. From (8.1) it follows that the rolling friction force is inversely proportional to the radius of the rolling body.

Liquid (viscous) is the friction between a solid and a liquid or gaseous medium or its layers.

where is the momentum of the system. Thus, the time derivative of the momentum of a mechanical system is equal to the geometric sum of the external forces acting on the system.

The last expression is law of conservation of momentum: The momentum of a closed-loop system is conserved, that is, it does not change over time.

Center of mass(or center of inertia) of a system of material points is called an imaginary point WITH, the position of which characterizes the mass distribution of this system. Its radius vector is equal to

Where m i And r i- mass and radius vector, respectively i th material point; n- number of material points in the system; – mass of the system. Center of mass speed

Considering that pi = m i v i, a there is an impulse R systems, you can write

that is, the momentum of the system is equal to the product of the mass of the system and the speed of its center of mass.

Substituting expression (9.2) into equation (9.1), we obtain

that is, the center of mass of the system moves as a material point in which the mass of the entire system is concentrated and on which a force acts equal to the geometric sum of all external forces applied to the system. Expression (9.3) is law of motion of the center of mass.

In accordance with (9.2), it follows from the law of conservation of momentum that the center of mass of a closed system either moves rectilinearly and uniformly or remains stationary.

5) Moment of force F relative to a fixed point ABOUT is a physical quantity determined by the vector product of the radius vector r drawn from the point ABOUT exactly A application of force, force F(Fig. 25):

Here M - pseudovector, its direction coincides with the direction of translational motion of the right propeller as it rotates from r to F. Modulus of the moment of force

where a is the angle between r and F; r sina = l- the shortest distance between the line of action of the force and the point ABOUT -shoulder of strength.

Moment of force about a fixed axis z called scalar magnitude Mz, equal to the projection onto this axis of the vector M of the moment of force determined relative to an arbitrary point ABOUT given z axis (Fig. 26). Torque value M z does not depend on the choice of point position ABOUT on the z axis.

If the z axis coincides with the direction of the vector M, then the moment of force is represented as a vector coinciding with the axis:

We find the kinetic energy of a rotating body as the sum of the kinetic energies of its elementary volumes:

Using expression (17.1), we obtain

Where J z - moment of inertia of the body relative to the z axis. Thus, the kinetic energy of a rotating body

From a comparison of formula (17.2) with expression (12.1) for the kinetic energy of a body moving translationally (T=mv 2 /2), it follows that the moment of inertia is measure of body inertia during rotational motion. Formula (17.2) is valid for a body rotating around a fixed axis.

In the case of plane motion of a body, for example a cylinder rolling down an inclined plane without sliding, the energy of motion is the sum of the energy of translational motion and the energy of rotation:

Where m- mass of the rolling body; vc- speed of the body's center of mass; Jc- moment of inertia of a body relative to an axis passing through its center of mass; w- angular velocity of the body.

6) To quantitatively characterize the process of energy exchange between interacting bodies, the concept is introduced in mechanics work of force. If the body moves straight forward and it is acted upon by a constant force F, which makes a certain angle  with the direction of movement, then the work of this force is equal to the product of the projection of the force F s to the direction of movement ( F s= F cos), multiplied by the displacement of the point of application of the force:

In the general case, the force can change both in magnitude and direction, so formula (11.1) cannot be used. If, however, we consider the elementary displacement dr, then the force F can be considered constant, and the movement of the point of its application can be considered rectilinear. Elementary work force F on displacement dr is called scalar magnitude

where  is the angle between vectors F and dr; ds = |dr| - elementary path; F s - projection of vector F onto vector dr (Fig. 13).

Work of force on the trajectory section from the point 1 to the point 2 equal to the algebraic sum of elementary work on individual infinitesimal sections of the path. This sum is reduced to the integral

To characterize the rate of work done, the concept is introduced power:

During time d t force F does work Fdr, and the power developed by this force at a given time

i.e., it is equal to the scalar product of the force vector and the speed vector with which the point of application of this force moves; N- magnitude scalar.

Unit of power - watt(W): 1 W is the power at which 1 J of work is performed in 1 s (1 W = 1 J/s).

Kinetic energy of a mechanical system is the energy of mechanical movement of this system.

Force F, acting on a body at rest and causing it to move, does work, and the energy of a moving body increases by the amount of work expended. Thus, work d A force F on the path that the body has passed during the increase in speed from 0 to v goes to increase kinetic energy d T bodies, i.e.

Using Newton's second law and multiplying by the displacement dr we get

Potential energy- mechanical energy of a system of bodies, determined by their relative position and the nature of the interaction forces between them.

Let the interaction of bodies be carried out through force fields (for example, a field of elastic forces, a field of gravitational forces), characterized by the fact that the work done by the acting forces when moving a body from one position to another does not depend on the trajectory along which this movement occurred, and depends only on the start and end positions. Such fields are called potential, and the forces acting in them are conservative. If the work done by a force depends on the trajectory of the body moving from one point to another, then such a force is called dissipative; an example of this is the force of friction.

The specific form of the function P depends on the nature of the force field. For example, the potential energy of a body of mass T, raised to a height h above the Earth's surface is equal to

where is the height h is counted from the zero level, for which P 0 =0. Expression (12.7) follows directly from the fact that potential energy is equal to the work done by gravity when a body falls from a height h to the surface of the Earth.

Since the origin is chosen arbitrarily, the potential energy can have a negative value (kinetic energy is always positive!). If we take the potential energy of a body lying on the surface of the Earth as zero, then the potential energy of a body located at the bottom of the mine (depth h"), P= -mgh".

Let's find the potential energy of an elastically deformed body (spring). The elastic force is proportional to the deformation:

Where Fx pack p - projection of elastic force onto the axis X;k- elasticity coefficient(for a spring - rigidity), and the minus sign indicates that Fx UP p is directed in the direction opposite to the deformation x.

According to Newton's third law, the deforming force is equal in magnitude to the elastic force and directed oppositely to it, i.e.

Elementary work d A, done by force Fx at infinitesimal deformation d x, equal to

a full job

goes to increase the potential energy of the spring. Thus, the potential energy of an elastically deformed body

The potential energy of a system is a function of the state of the system. It depends only on the configuration of the system and its position in relation to external bodies.

When the system transitions from the state 1 to any state 2

that is, the change in the total mechanical energy of the system during the transition from one state to another is equal to the work done by external non-conservative forces. If there are no external non-conservative forces, then from (13.2) it follows that

d ( T+P) = 0,

that is, the total mechanical energy of the system remains constant. Expression (13.3) is law of conservation of mechanical energy: in a system of bodies between which only conservative forces act, the total mechanical energy is conserved, that is, it does not change with time.

Linear movement, linear speed, linear acceleration.

Moving(in kinematics) - a change in the location of a physical body in space relative to the selected reference system. The vector characterizing this change is also called displacement. It has the property of additivity. The length of the segment is the displacement module, measured in meters (SI).

You can define movement as a change in the radius vector of a point: .

The displacement module coincides with the distance traveled if and only if the direction of displacement does not change during movement. In this case, the trajectory will be a straight line segment. In any other case, for example, with curvilinear motion, it follows from the triangle inequality that the path is strictly longer.

Vector D r = r -r 0 drawn from the initial position of the moving point to its position at a given time (increment of the radius vector of the point over the considered period of time) is called moving.

During rectilinear motion, the displacement vector coincides with the corresponding section of the trajectory and the displacement module |D r| equal to the distance traveled D s.
Linear speed of a body in mechanics

Speed

To characterize the motion of a material point, a vector quantity is introduced - speed, which is defined as rapidity movement and his direction at a given moment in time.

Let a material point move along some curvilinear trajectory so that at the moment of time t it corresponds to the radius vector r 0 (Fig. 3). For a short period of time D t the point will go along the path D s and will receive an elementary (infinitesimal) displacement Dr.

Average speed vector is the ratio of the increment Dr of the radius vector of a point to the time interval D t:

The direction of the average velocity vector coincides with the direction of Dr. With an unlimited decrease in D t the average speed tends to a limiting value called instantaneous speed v:

Instantaneous speed v, therefore, is a vector quantity equal to the first derivative of the radius vector of the moving point with respect to time. Since the secant in the limit coincides with the tangent, the velocity vector v is directed tangent to the trajectory in the direction of motion (Fig. 3). As D decreases t path D s will increasingly approach |Dr|, so the absolute value of the instantaneous velocity

Thus, the absolute value of the instantaneous speed is equal to the first derivative of the path with respect to time:

At uneven movement - the module of instantaneous speed changes over time. In this case, we use the scalar quantity b vñ - average speed uneven movement:

From Fig. 3 it follows that á vñ> |ávñ|, since D s> |Dr|, and only in the case of rectilinear motion

If expression d s = v d t(see formula (2.2)) integrate over time ranging from t before t+D t, then we find the length of the path traveled by the point in time D t:

When uniform motion the numerical value of the instantaneous speed is constant; then expression (2.3) will take the form

The length of the path traveled by a point during the period of time from t 1 to t 2, given by the integral

Acceleration and its components

In the case of uneven movement, it is important to know how quickly the speed changes over time. A physical quantity characterizing the rate of change in speed in magnitude and direction is acceleration.

Let's consider flat movement, those. a movement in which all parts of a point’s trajectory lie in the same plane. Let the vector v specify the speed of the point A at a point in time t. During time D t the moving point has moved to position IN and acquired a speed different from v both in magnitude and direction and equal to v 1 = v + Dv. Let's move the vector v 1 to the point A and find Dv (Fig. 4).

Medium acceleration uneven movement in the range from t before t+D t is a vector quantity equal to the ratio of the change in speed Dv to the time interval D t

Instant acceleration and (acceleration) of a material point at the moment of time t there will be a limit of average acceleration:

Thus, acceleration a is a vector quantity equal to the first derivative of speed with respect to time.

Let us decompose the vector Dv into two components. To do this from the point A(Fig. 4) in the direction of velocity v we plot the vector equal in absolute value to v 1 . Obviously, the vector , equal to , determines the change in speed over time D t modulo: . The second component of the vector Dv characterizes the change in speed over time D t in direction.

Tangential and normal acceleration.

Tangential acceleration- acceleration component directed tangentially to the motion trajectory. Coincides with the direction of the velocity vector during accelerated motion and in the opposite direction during slow motion. Characterizes the change in speed module. It is usually designated or (, etc. in accordance with which letter is chosen to denote acceleration in general in this text).

Sometimes tangential acceleration is understood as the projection of the tangential acceleration vector - as defined above - onto the unit vector of the tangent to the trajectory, which coincides with the projection of the (total) acceleration vector onto the unit tangent vector, that is, the corresponding expansion coefficient in the accompanying basis. In this case, not a vector notation is used, but a “scalar” one - as usual for the projection or coordinates of a vector - .

The magnitude of tangential acceleration - in the sense of the projection of the acceleration vector onto a unit tangent vector of the trajectory - can be expressed as follows:

where is the ground speed along the trajectory, coinciding with the absolute value of the instantaneous speed at a given moment.

If we use the notation for the unit tangent vector, then we can write the tangential acceleration in vector form:

Conclusion

The expression for tangential acceleration can be found by differentiating with respect to time the velocity vector, represented in terms of the unit tangent vector:

where the first term is the tangential acceleration, and the second is the normal acceleration.

Here we use the notation for the unit normal vector to the trajectory and - for the current length of the trajectory (); the last transition also uses the obvious

and, from geometric considerations,

Centripetal acceleration(normal)- part of the total acceleration of a point, due to the curvature of the trajectory and the speed of movement of the material point along it. This acceleration is directed towards the center of curvature of the trajectory, which is what gives rise to the term. Formally and essentially, the term centripetal acceleration generally coincides with the term normal acceleration, differing rather only stylistically (sometimes historically).

Particularly often we talk about centripetal acceleration when we are talking about uniform motion in a circle or when motion is more or less close to this particular case.

Elementary formula

where is the normal (centripetal) acceleration, is the (instantaneous) linear speed of movement along the trajectory, is the (instantaneous) angular velocity of this movement relative to the center of curvature of the trajectory, is the radius of curvature of the trajectory at a given point. (The connection between the first formula and the second is obvious, given).

The expressions above include absolute values. They can be easily written in vector form by multiplying by - a unit vector from the center of curvature of the trajectory to a given point:

These formulas are equally applicable to the case of motion with a constant (in absolute value) speed and to an arbitrary case. However, in the second, one must keep in mind that centripetal acceleration is not the full acceleration vector, but only its component perpendicular to the trajectory (or, what is the same, perpendicular to the instantaneous velocity vector); the full acceleration vector then also includes a tangential component (tangential acceleration), the direction coinciding with the tangent to the trajectory (or, what is the same, with the instantaneous speed).

Conclusion

The fact that the decomposition of the acceleration vector into components - one along the tangent to the vector trajectory (tangential acceleration) and the other orthogonal to it (normal acceleration) - can be convenient and useful is quite obvious in itself. This is aggravated by the fact that when moving at a constant speed, the tangential component will be equal to zero, that is, in this important particular case, only the normal component remains. In addition, as can be seen below, each of these components has clearly defined properties and structure, and normal acceleration contains quite important and non-trivial geometric content in the structure of its formula. Not to mention the important particular case of motion in a circle (which, moreover, can be generalized to the general case with virtually no changes).